Quantity/Volume Calculators for Concrete
(Metric)

These calculators are intended to allow you to calculate the metric volume of concrete required for paths etc.

Three calculators are provided, the first for rectangle areas of concrete, the second for circular areas and the final one for triangular areas.

Rectangular calculator.
Circular calculator.
Triangular calculator.

See also our imperial calculators.

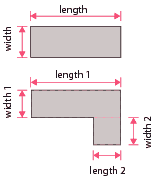
Rectangular metric volume calculator

Enter the dimensions of the rectangle to be concreted:



You will need approximately.....

 

Note: This is the volume of the work space and does not take into account the concrete 'shrinkage'

Squares and rectangles

For a simple rectangle, just use the length and width with the thickness in the calculator left.

For irregular, basically square areas,

  • Breakdown the area into a number of simple rectangular areas.
  • Workout the volume for each area separately.
  • And finally add the separate volumes together.

Circular metric volume calculator

Enter the radius of the circle to be concreted:


You will need approximately.....

Note: This is the volume of the work space and does not take into account the concrete 'shrinkage'
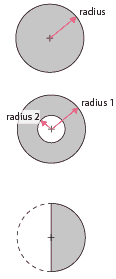Circles

For a simple circle, just use the radius with the thickness in the calculator left.

For a ring:

  • Use the calculator to work out the volume for both the inner and outer circles.
  • Take the volume for the inner circle away from the volume for the outer circle and you will be left with the volume of the ring.

For part of a circle, calculate the volume for the full circle and then adjust for the part required - i.e. halve for a half circle, quarter for a quarter circle.

Triangular metric volume calculator

Enter the dimensions of the triangular areas to be concreted:



You will need approximately.....

 

Note: This is the volume of the work space and does not take into account the concrete 'shrinkage'

triangular shape

For triangular shapes, work out the height and base of the triangle and use with the thickness in the calculator left.

The 'height' is the distance between an angle and the opposite side where a line meets the side at right angles. The side it meets is the 'base'.